How to Calculate the Volume of a Hemisphere?
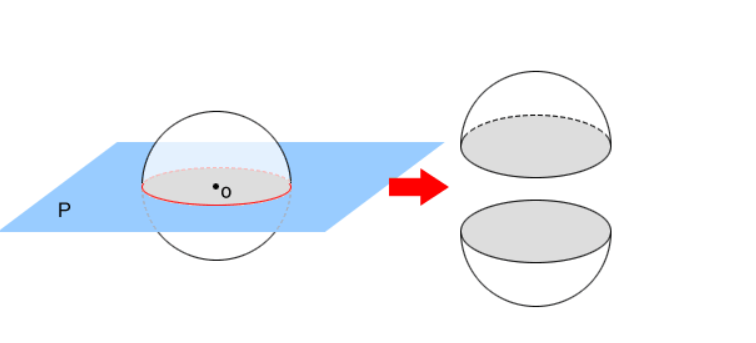
Grade 7 students learn about the volume of a hemisphere in their math class. Since a hemisphere is a half sphere, you can calculate the volume of a hemisphere by first finding the volume of a sphere and then dividing it by two. The volume of a sphere is calculated using the following formula:
V = 4/3 x π x r3
where V is the volume of the sphere, π is 3.14 (pi), and r is the radius of the sphere.
To find the volume of a hemisphere, you would first need to find the radius of the sphere. The radius of a sphere is equal to half of its diameter. Once you have the radius, plug it into the formula and solve for V. Then, divide the volume of the sphere by two to get the volume of the hemisphere.
Here is an example:
Find the volume of a hemisphere with a diameter of 10 cm.
First, find the radius of the sphere. The radius is equal to half of the diameter, so the radius would be 5 cm.
Next, plug the values into the formula and solve for V.
V = 4/3 x 3.14 x 5 cm3
V = 4.18 x 5 cm3
V = 20.9 cm3
Finally, divide the volume of the sphere by two to find the volume of the hemisphere.
20.9 cm3 ÷ 2 = 10.45 cm3
The volume of the hemisphere is 10.45 cm3.
You can also directly calculate the volume of the hemisphere by using the formula:
v (Volume of a hemisphere) = V (Volume of a sphere)/2
v = (4/3 x π x r3)/2
v = 2/3 x π x r3
If you use this formula, then you could solve the above question as follows:
v = 2/3 x 3.14 x 5 cm3
v = 10.45 cm3
Are you curious to know why the formula for the volume of a sphere is 4/3πr3?
Online math tutors at CrunchGrade love inquisitive students who want to understand the concepts behind the formulas.
Derivation of the volume of sphere formula will need an understanding of integration or the relationship between volumes of a cone, cylinder and sphere. While the derivation of the volume of a sphere is above this grade level, here is a quick explanation.
Grade 7 students can learn about the relationship between volumes of a cone, cylinder and sphere in this online math lesson using a practical experiment.
The volume of a sphere is equal to the volume of two cones. You can see that by taking a sphere with radius r (say 5 cm) and height h (which is 2r), and a cone with the same radius and height. You can fill a cone with water and pour it into the hollow sphere. You will see that if you do this twice, the sphere will fill up completely with water.
Now, the volume of the cone is 1/3 x πr2h. Since the volume of the sphere is twice the volume of the cone, it will be:
V = 2 x 1/3 x πr2h = 2/3 x πr2h
Since h = 2r,
V = 2/3 x πr2 x 2r = 4/3 x π x r3
Since the volume of a hemisphere is half of the volume of a sphere, its formula is:
v = (4/3 x π x r3)/2 = 2/3 x π x r3
Contact us for a free trial session to explore math concepts in greater depth with one of our expert math tutors online.
